My second assignment on groups was marked and returned. As predicted, there's a lot to take away from this.
Basic Groups
At this level it seems it's not sufficient to use notation like 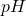 ,
, 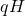 and
and 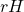 to refer to subgroups. In my head, I know what the binary operator of these subgroups is but for the benefit of the reader the (better) convention is to be explicit with the binary operator:
to refer to subgroups. In my head, I know what the binary operator of these subgroups is but for the benefit of the reader the (better) convention is to be explicit with the binary operator: 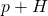 ,
, 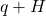 , and
, and 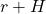 specifically.
specifically.
Definition check: a cyclic group is just a group that contains a generator that generates the whole group. It doesn't have to "cycle" back to the beginning of the ordered set. eg, infinite sets can form a cyclic group under addition.
Mistakes in basic proofs. This one is a classic. With 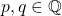 , I had to prove
, I had to prove 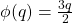 was onto. My answer:
was onto. My answer:
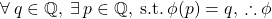
Which I was all smug about because it looks pretty. But this is just the definition of the function being onto and I'd neglected to state the actual 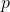 that always exists. Namely
that always exists. Namely 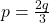 .
.
Group Classification
Group decompositions can't be written as 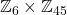 . It needs to be written as
. It needs to be written as 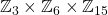 . 3 and 15 are not coprime, so cannot be combined.
. 3 and 15 are not coprime, so cannot be combined.
Finite Groups
As a part of a group presentation, there are kind of identities like 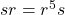 . However, for the most part in your answer, especially when you're talking directly about elements being in your group, they must always be in the form
. However, for the most part in your answer, especially when you're talking directly about elements being in your group, they must always be in the form 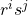 (with
(with  first).
first).
In being asked to deduce an isomorphism, I heavy-handedly defined both groups (as they were small) and then also stated a function explicitly that would define the isomorphism. Apparently it's just enough to state that both groups were of order 2. Therefore they're isomorphic.
I need to become a lot more familiar with the concept of centres of a group. To show that a subgroup is the centre of its parent group, I needed to show that the subgroups elements were centres of the subgroup. I was was just trying to wing it, and although I essentially got the answer right, I showed I had a complete misunderstanding of centres.
